As a follow-up to my last post on extraordinary claims require extraordinary evidence (ECREE), I’m going to respond to a blog post by T. Kurt Jaros. As in my previous posts,let B represent our background information; E represent our evidence to be explained; H be an explanatory hypothesis, and ~H be the falsity of H.

Objection: “The trouble with the first part [of the phrase (“extraordinary claims”)] is that we’re not quite certain what precisely are extraordinary claims. Again, whether a claims is extraordinary or not depends entirely upon what you know and believe.  Put nicely above, if you know nothing, then everything is an extraordinary claim.”

Reply: According to the Bayesian interpretation of ECREE, the relevant probabilities are to be understood as epistemic probabilities (as opposed to the classical, logical, or other interpretations of probability). So the objector is correct that the Bayesian interpretation is inherently subjective in the sense that it depends entirely upon what a person knows and believes. So what? It doesn’t follow that we can’t figure out what are extraordinary claims.

Again, as I wrote in my previous posts on ECREE, an “extraordinary claim” is an explanatory hypothesis which is extremely improbable, conditional upon background information alone, i.e., Pr(H | B) < x, where x can be any real number between 0 and 1. I proposed that x be some real number that is very much less than 0.5, but the value of X ultimately doesn’t matter. In terms of calculating the final probability of H, Pr(H | E & B), we use the same formula–BT–regardless of whether H is an extraordinary claim.

Thus, even if it were true that, “If you know nothing, then everything is an extraordinary claim,” absolutely nothing of significance would follow from this fact on the Bayesian interpretation of ECREE. As we shall we see below, we use the same formula for both ordinary and extraordinary claims to determine the evidence required to establish a high final probability for a claim.

 

Objection: “The second part of the phrase [“extraordinary evidence”] is also troublesome.  For what precisely counts as extraordinary evidence?  Any thing that could be brought forth as “extraordinary evidence” would, in actuality, just be ordinary evidence. Evidence is simply the available facts or data about any given proposition.  Therefore, referring to some evidence as “extraordinary” is redundant.  It also is subjective.  Person A may think that evidence x categorizes as extraordinary evidence but person B may just consider it as ordinary evidence. So once again, how are we to define what qualifies as extraordinary?  Like the first part of the phrase, the second part seems to lack clear understanding of what constitutes as extraordinary evidence.”

Reply: It is a truism that, according to the epistemic interpretation of probability, probabilities are subjective. It doesn’t follow, however, that “extraordinary evidence” is hard to define. Applying BT, we can define “extraordinary evidence” as any evidence E that makes the following inequality true:

whenever H is considered an “extraordinary claim,” i.e., Pr(H | B) < x, where x can be any real number between 0 and 1. Now consider the definition of “ordinary evidence.” Ordinary evidence is any evidence E that makes the following inequality true: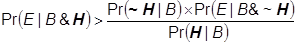

whenever H is not considered an “extraordinary claim.”

Notice that the inequalities are the same for both ordinary and extraordinary evidence. This might lead one to wonder, “Then why bother with the ECREE slogan at all?” The answer is this. ECREE emphasizes the common sense notion that the more implausible (i.e., antecedently improbable) we initially regard a claim prior to considering the evidence, the greater the evidence we will require to believe the claim. (In the jargon of Bayesianism, we might say that ECREE captures the common sense requirement that a hypothesis’s explanatory power is proportionally high enough to offset its prior improbability.) The Bayesian interpretation of ECREE, like BT itself, specifies the pattern of probability relations which must exist in order for a claim to have a high epistemic final probability.[1]

Notes

[1] I owe this point to Robert Greg Cavin.