The Unmoved Mover Argument – Part 6: More on Something Exists
In his book When Skeptics Ask (hereafter: WSA), Norman Geisler presents his general version of a Thomist Cosmological Argument (hereafter: TCA). The first premise of Geisler’s TCA is this:
1. Finite, changing things exist. (WSA, p.18)
Geisler provides a very brief argument in support of (1) in WSA. In Part 4 of this series I showed that Geisler’s brief argument in support of (1) was a stinking philosophical TURD. It FAILS utterly and completely to support ANY part of premise (1).
In Part 5 of this series I clarified and analyzed a longer and more sophisticated argument by Geisler in support of just one part of premise (1) of TCA, an argument that is found in his much older book Philosophy of Religion (hereafter: PoR). This longer argument only supports the simple and obviously true claim that “Something exists”. Geisler provides further arguments in PoR for the claim that there are finite, changing things. But I will get into those further arguments in later posts of this series.
There were eight explicit statements in Geisler’s longer argument, but in attempting to re-construct the logic of his argument it became apparent that the argument contained several logical gaps which needed to be filled by making explicit various unstated assumptions in the argument. My previous diagram of the resulting re-construction of Geisler’s argument contains seven clarified versions of Geisler’s original statements plus five additional assumptions, required to make the argument logically valid. Furthermore, when I evaluated a number of the initial sub-arguments in the overall argument, I discovered further logical gaps, and added three more assumptions to my re-construction of this argument.
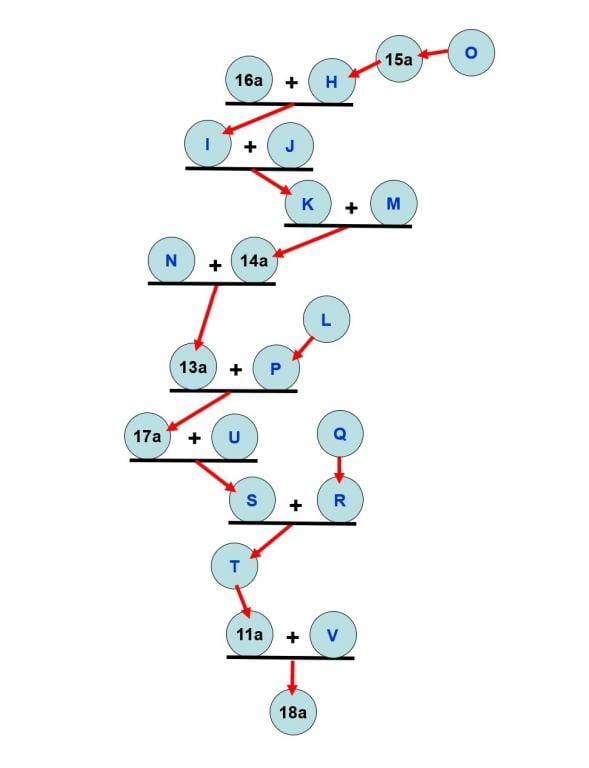
Here is the logical structure of Geisler’s longer and more complex argument in support of the claim that “Something exists”, including the three additional assumptions (the numbered circles represent explicit statements, and the lettered circles represent unstated assumptions that I made explicit to clarify the argument):
In my previous evaluation of this longer and more complex argument, I examined each inference and sub-argument, beginning with the inference from (15a) to (H), through the sub-argument with the inference from premises (N) and (14a) to premise (13a). In this post I will finish evaluating the final premises and inferences of this argument.
My evaluation of the argument so far is that it is clearly UNSUCCESSFUL even though each premise (so far) appears to be TRUE, and each inference (so far) appears to be VALID. The problem is that premise (J) is LESS obvious and LESS certain than the conclusion that “Something exists”, which makes premise (K) Less obvious and LESS certain than the conclusion, and the inference from (K) and (M) to (14a) is also not entirely obvious and certain, so by the time we get to premise (14a), that premise is clearly LESS obvious and LESS certain than the conclusion that “Something exists”. In the case of a deductive proof for a conclusion, the premises of the argument must all be MORE obvious and MORE certain than the conclusion in order for the argument to have any significance or value. So, this longer more complex argument by Geisler FAILS.
I will continue, nevertheless, to evaluate the rest of this longer argument to determine if there are any more problems or weaknesses in the argument. The next sub-argument to evaluate is this one:
13a. Any attempt by a person to deny his/her own existence is self-defeating.
L. When a person denies the existence of everything, that person is denying his/her own existence.
THEREFORE:
17a. All attempts by a person to deny the existence of everything are self-defeating.
I accept premise (13a) as TRUE, because it appears to be a VALID deductive inference from (N) and (14a) which I accept as TRUE, although (14a) is clearly LESS obvious and LESS certain than the conclusion that “Something exists”. I accept premise (L) as being obviously TRUE. So, the remaining question is whether the inference is deductively VALID. I believe the inference is VALID, but it is not formally VALID. That is mainly because of the way I formulated the unstated assumption (L). I could have formulated a supplementary premise in a way that would have made this inference formally VALID:
13a. Any attempt by a person to deny his/her own existence is self-defeating.
P. IF any attempt by a person to deny his/her own existence is self-defeating, THEN all attempts by a person to deny the existence of everything are self-defeating.
THEREFORE:
17a. All attempts by a person to deny the existence of everything are self-defeating.
The addition of premise (P) turns this sub-argument into a formally VALID deductive inference (called a modus ponens). The problem is that (P) is not as clearly and obviously true as (L). I suppose that we can take (L) to be a reason supporting (P), making the truth of (P) more clear and obvious than it would otherwise be, and then (P) makes the sub-argument formally deductively VALID. I believe that (P) is TRUE, but there is a complexity to (P) that requires some thinking to evaluate it’s truth, and that makes it LESS obvious and LESS certain than the conclusion that “Something exists”. So, this appears to be a THIRD step-down in the degree of obviousness and certainty that this argument provides relative to the obviousness and certainty of the conclusion (prior to the giving of the argument).
The next inference in the argument is from (17a) to (11a):
17a. All attempts by a person to deny the existence of everything are self-defeating.
THEREFORE:
11a. It is undeniable that something exists.
There are some missing steps of logic here. The denial of “the existence of everything”, implies the claim that “nothing exists”, so the FALSEHOOD of the denial of “the existence of everything” implies the FALSEHOOD of the claim that “nothing exists”, and if the claim that “nothing exists” is FALSE, then that implies that the claim “something exists” is TRUE. So we can get from the FALSEHOOD of the denial of “the existence of everything” to the conclusion that “something exists” is TRUE.
However, this inference from (17a) to (11a) is not quite that simple. It talks about “attempts” by a person “to deny the existence of everything”, not about the denial itself. It talks about such “attempts” being “self-defeating” as opposed to being FALSE. Furthermore, the conclusion is not that “something exists”, but that it is “undeniable” that something exists.
In any case, this inference is NOT a formally VALID inference. There may be a chain of deductive inferences that could link (17a) to (11a), but it is not at all clear what the steps of reasoning would be here. The main problem is going from the concept of a denial being “self-defeating” to the concept of a claim being “undeniable”. Geisler has once again introduced a new term in (11a), a term that does NOT appear in the previous premises of the argument. That messes up the logic of the argument.
Once again, we need a premise that clarifies or defines the new term that Geisler has thrown into the argument: “undeniable”. I think Geisler was assuming that if the denial of a claim X is self-defeating, then claim X is “undeniable”:
Q. IF the denial of claim X is self-defeating, THEN claim X is undeniable.
THEREFORE:
R. IF the denial of the claim “Something exists” is self-defeating, THEN the claim “Something exists” is undeniable
S. The denial of the claim “Something exists” is self-defeating.
THEREFORE:
T. The claim “Something exists” is undeniable.
THEREFORE:
11a. It is undeniable that something exists.
The final inference from (T) to (11a) is still not formally VALID, but it seems so clearly to be logically implied by (T), that I will accept this inference as being a VALID deductive inference. So, this is how I would repair the inference from (17a) to (11a), except that we still need to show that (17a) logically implies the additional premise (S) above.
I take it that (Q) is a partial stipulative definition of “undeniable”, and is thus TRUE, and I take it that (Q) logically implies (R), so (R) is also clearly and obviously TRUE. So, the only thing that remains questionable in this revised sub-argument is whether (17a) logically implies premise (S):
17a. All attempts by a person to deny the existence of everything are self-defeating.
U. To deny the existence of everything is the same as to deny the claim “Something exists”.
THEREFORE:
S. The denial of the claim “Something exists” is self-defeating.
Premise (U) is obviously and certainly TRUE, and the inference from (17a) and (U) to (S) is VALID, so this sub-argument is SOUND and acceptable.
The final inference in Geisler’s argument is this one:
11a. It is undeniable that something exists.
THEREFORE:
18a. Something exists.
As it stands, this inference is NOT formally VALID. In order to determine whether the inference from (11a) to (18a) is deductively VALID, we need to understand the logical implications of the term “undeniable” in premise (11a). If a claim is “undeniable”, does that necessarily mean that the claim is TRUE? We need a clarification or definition of “undeniable” that allows us to bridge the logical gap between premise (11a) and the conclusion (18a):
11a. It is undeniable that something exists.
V. IF claim X is undeniable, THEN claim X is true.
THEREFORE:
18a. Something exists.
My first inclination is to say that we simply don’t know whether premise (V) is true or false, because we simply don’t know what it MEANS for a claim to be “undeniable”. But in evaluating an earlier part of this argument, we had to supply a premise that partially defined this term, in order to make one of the inferences in this argument logically valid. So, here is the premise that we added in order to repair a logical gap in Geisler’s reasoning:
Q. IF the denial of claim X is self-defeating, THEN claim X is undeniable.
But because this was only a partial definition, and because it only stated a sufficient condition for a claim being “undeniable”, this will be of no help for our evaluation of premise (V).
We need to know the logical implications of a claim being “undeniable”, which means we need to know the NECESSARY CONDITIONS for a claim being “undeniable”, not the sufficient conditions. Specifically, we need to determine whether a claim must be TRUE in order for it to be “undeniable”. But Geisler gave us no clarification or definition of the term “undeniable”, and the argument up to this point only assumes (Q) which provides us with just a sufficient condition.
Recall that we get to the conclusion that “Something exists” is “undeniable” on the basis of the previous claim that the denial of the claim “Something exists” is “self-defeating”:
R. IF the denial of the claim “Something exists” is self-defeating, THEN the claim “Something exists” is undeniable
S. The denial of the claim “Something exists” is self-defeating.
THEREFORE:
T. The claim “Something exists” is undeniable.
So, perhaps if we understand what it MEANS for the denial of a claim to be “self-defeating” we could determine whether all such claims must be TRUE.
It is now becoming clear to me that there is an important distinction that we need to keep in mind between “the negation of claim X” and “the denial of claim X by person P”. On the one hand, the denial of the claim “Something exists” by a person P is “self-defeating” because person P is something that exists. But this contrasts with the negation of the claim that “Something exists”, which is “It is NOT the case that something exists”, which means the same as “Nothing exists”.
The claim that “Nothing exists,” as Geisler himself points out, is a logical possibility. It is logically possible for it to be the case that nothing exists. So, there is no intrinsic logical self-contradiction involved in the statement “Nothing exists”. The self-defeating aspect of the claim “Nothing exists” occurs only when a PERSON affirms this claim.
In other words, the “undeniable” character of the claim “Something exists” has to do with the existence of a PERSON who either affirms or denies that “Something exists”. It has nothing to do with the intrinsic logic of the statement “Something exists”.
Therefore, even if we grant the assumption that the claim “Something exists” is “undeniable”, it remains logically possible for the statement “Something exists” to be FALSE. Therefore, the additional premise required to make the final inference of Geisler’s argument logically VALID is a FALSE premise:
11a. It is undeniable that something exists.
V. IF claim X is undeniable, THEN claim X is true.
THEREFORE:
18a. Something exists.
It is logically possible for the antecedent of (V) to be TRUE, and yet for the consequent to be FALSE. Premise (V) is thus FALSE, so the final sub-argument in Geisler’s long and complex argument for the conclusion that “Something exists” is an UNSOUND argument! Therefore, Geisler’s argument for the conclusion “Something exists” clearly and definitely FAILS.
CONCLUSION
This argument is very much like a “Shaggy Dog” joke, where the punchline is really stupid, or where the person telling the joke forgets the punchline after they are already five minutes into telling the joke story-line.
Here is my final diagram of the logical structure of Geisler’s argument for the simple and obviously true conclusion that “Something exists”:
This argument consists of twenty-two statements, seven of which were explicitly asserted by Geisler (the statements identified with numbers), and fifteen of which were unstated assumptions (the statements identified with letters), plus thirteen inferences (indicated by red arrows). It should come as no surprise that, given the length and complexity of this argument, some of the premises and inferences in this argument are LESS obvious and/or LESS certain than the obviously true conclusion that “Something exists”.
The final sub-argument involves an unstated assumption that is clearly FALSE, so this final sub-argument is UNSOUND, which means the whole argument FAILS. But there were also earlier problems with the argument that also make it so this argument FAILS, even if all of the premises of the argument were accepted as TRUE and all of the inferences were accepted as VALID.
Some of the premises and inferences were LESS obvious and LESS certain than the conclusion of the argument “Something exists”, and with a deductive proof, ALL of the premises and inferences in the argument need to be MORE obvious and MORE certain than the conclusion of the argument (or at least AS obvious and AS certain as the conclusion). So, even without the FALSE premise in the final sub-argument, this argument still would have FAILED to provide a legitimate proof of the conclusion that “Something exists.”
Specifically, the sub-argument that infers (14a) from premises (K) and (M) has two problems that make it so that premise (14a) is definitely LESS obvious and LESS certain than the claim that “Something exists”.
The truth of (K) is based in part on the previous premise (J) which is LESS obvious and LESS certain than “Something exists”, making (K) similarly LESS obvious and LESS certain than “Something exists”. Furthermore the VALIDITY of the inference from (K) and (M) to (14a) is LESS obvious and LESS certain than the truth of the claim “Something exists”. So, the sub-argument supporting (14a) clearly FAILS to make (14a) anything other than LESS obvious and LESS certain than the conclusion that “Something exists”. Therefore, the problems with this sub-argument for (14a) are also enough all by themselves to make Geisler’s overall argument here FAIL.